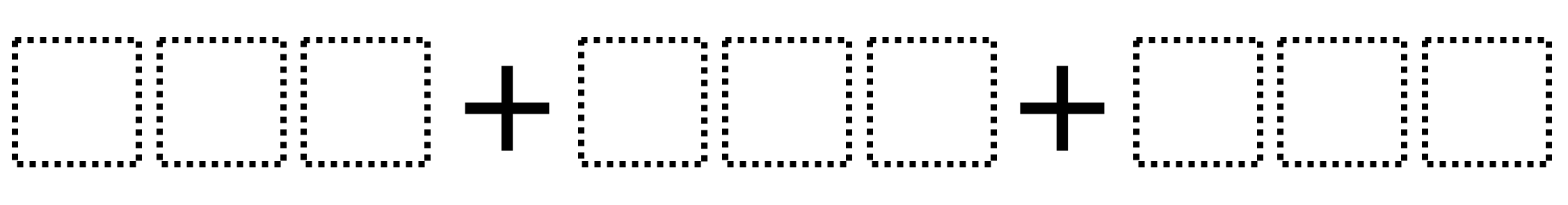
Directions: Using the digits 1 to 9 exactly one time each, place a digit in each box to make the sum as close to 1000 as possible.
Hint
How do you know you can’t get any closer to 1000? What should be true about the hundreds places of your three numbers? How do the tens places affect your answer?
Answer
Lots of answers will get you 999. One would be 247 + 563 + 189.
Is exactly 1000 even possible? Here’s a Geogebra tool you can use to check your answer.
Is exactly 1000 even possible? Here’s a Geogebra tool you can use to check your answer.
Source: John Ulbright and Robert Kaplinsky
 Open Middle®
Open Middle®



One would be 189+247+563
Hi, I’m a 1st time visitor. We are just really getting started recording various levels of DOK questions within our common lessons. I found your site via a Goggle search. However, I have a question. How can I confirm if a question is a DOK 2 or 3? They do not seem to be identified. Are the levels noted somewhere?
Thanks,
Laura
Hi Laura. You can see it in the gray tags area just above the comments section. This problem is DOK 3.
You are yo sissy
173 248 569
What is DOK? Do you mean DORK?
600+300+100=1000
DDDDDDDDDDDDDDEEEEEEEEEEEEEEEVVVVVVVVVVVNNNNNNNNNNNN YO SISSY
Devn, that isn’t true. You cannot use 0s.
176+534+289=999
152+368+479=999
583+167+249=999
To pique student interest and involvement, I put a leaderboard on the classroom whiteboard. This showed first, second, third and fourth places of who in the class could get closest to 1000. It was great to see so many students taking up the challenge to get first place.
This is you ” Blah blah blah blah blah blah”
By the way, where do I find the DOK that goes with this resource? I’m having trouble finding it on the website.
The DOK is listed in the tags just below the question and just above the comments section.
What is DOK? Do you mean DORK?
I was wondering if we could extend the problem by adding closest to 1,000 meaning above or below it. It doesn’t specify, but wondered if that would be another option to use?
234+324+401=959
152 + 528 + 329 = 999
250 + 250 + 500 would get 1,000.
cant use 0 and cant use the same number more than once
169+452+378=999
169+458+372=999
169+472+358=999
169+478+352=999
169+543+287=999
169+547+283=999
169+583+247=999
169+587+243=999
172+358+469=999
172+359+468=999
172+368+459=999
172+369+458=999
172+458+369=999
172+459+368=999
172+468+359=999
172+469+358=999
174+236+589=999
174+239+586=999
174+286+539=999
174+289+536=999
174+536+289=999
174+539+286=999
174+586+239=999
174+589+236=999
175+326+498=999
175+328+496=999
175+396+428=999
175+398+426=999
175+426+398=999
175+428+396=999
175+496+328=999
175+498+326=999
176+234+589=999
176+239+584=999
176+284+539=999
176+289+534=999
176+325+498=999
176+328+495=999
176+395+428=999
176+398+425=999
176+425+398=999
176+428+395=999
176+495+328=999
176+498+325=999
176+534+289=999
176+539+284=999
176+584+239=999
176+589+234=999
178+325+496=999
178+326+495=999
178+352+469=999
178+359+462=999
178+362+459=999
178+369+452=999
178+395+426=999
178+396+425=999
178+425+396=999
178+426+395=999
178+452+369=999
178+459+362=999
178+462+359=999
178+469+352=999
178+495+326=999
178+496+325=999
179+234+586=999
179+236+584=999
179+284+536=999
179+286+534=999
179+352+468=999
179+358+462=999
179+362+458=999
179+368+452=999
179+452+368=999
179+458+362=999
179+462+358=999
179+468+352=999
179+534+286=999
179+536+284=999
179+584+236=999
179+586+234=999
183+247+569=999
183+249+567=999
183+267+549=999
183+269+547=999
183+547+269=999
183+549+267=999
183+567+249=999
183+569+247=999
184+236+579=999
184+239+576=999
184+276+539=999
184+279+536=999
184+536+279=999
184+539+276=999
184+576+239=999
184+579+236=999
186+234+579=999
186+239+574=999
186+274+539=999
186+279+534=999
186+534+279=999
186+539+274=999
186+574+239=999
186+579+234=999
187+243+569=999
187+249+563=999
187+263+549=999
187+269+543=999
187+543+269=999
187+549+263=999
187+563+249=999
187+569+243=999
189+234+576=999
189+236+574=999
189+243+567=999
189+247+563=999
189+263+547=999
189+267+543=999
189+274+536=999
189+276+534=999
189+534+276=999
189+536+274=999
189+543+267=999
189+547+263=999
189+563+247=999
189+567+243=999
189+574+236=999
189+576+234=999
194+237+568=999
194+238+567=999
194+267+538=999
194+268+537=999
194+537+268=999
194+538+267=999
194+567+238=999
194+568+237=999
195+326+478=999
195+328+476=999
195+376+428=999
195+378+426=999
195+426+378=999
195+428+376=999
195+476+328=999
195+478+326=999
196+325+478=999
196+328+475=999
196+375+428=999
196+378+425=999
196+425+378=999
196+428+375=999
196+475+328=999
196+478+325=999
197+234+568=999
197+238+564=999
197+264+538=999
197+268+534=999
197+534+268=999
197+538+264=999
197+564+238=999
197+568+234=999
198+234+567=999
198+237+564=999
198+264+537=999
198+267+534=999
198+325+476=999
198+326+475=999
198+375+426=999
198+376+425=999
198+425+376=999
198+426+375=999
198+475+326=999
198+476+325=999
198+534+267=999
198+537+264=999
198+564+237=999
198+567+234=999
234+167+598=999
234+168+597=999
234+176+589=999
234+179+586=999
234+186+579=999
234+189+576=999
234+197+568=999
234+198+567=999
234+567+198=999
234+568+197=999
234+576+189=999
234+579+186=999
234+586+179=999
234+589+176=999
234+597+168=999
234+598+167=999
236+174+589=999
236+179+584=999
236+184+579=999
236+189+574=999
236+574+189=999
236+579+184=999
236+584+179=999
236+589+174=999
237+164+598=999
237+168+594=999
237+194+568=999
237+198+564=999
237+564+198=999
237+568+194=999
237+594+168=999
237+598+164=999
238+164+597=999
238+167+594=999
238+194+567=999
238+197+564=999
238+564+197=999
238+567+194=999
238+594+167=999
238+597+164=999
239+174+586=999
239+176+584=999
239+184+576=999
239+186+574=999
239+574+186=999
239+576+184=999
239+584+176=999
239+586+174=999
243+167+589=999
243+169+587=999
243+187+569=999
243+189+567=999
243+567+189=999
243+569+187=999
243+587+169=999
243+589+167=999
247+163+589=999
247+169+583=999
247+183+569=999
247+189+563=999
247+563+189=999
247+569+183=999
247+583+169=999
247+589+163=999
249+163+587=999
249+167+583=999
249+183+567=999
249+187+563=999
249+563+187=999
249+567+183=999
249+583+167=999
249+587+163=999
263+147+589=999
263+149+587=999
263+187+549=999
263+189+547=999
263+547+189=999
263+549+187=999
263+587+149=999
263+589+147=999
264+137+598=999
264+138+597=999
264+197+538=999
264+198+537=999
264+537+198=999
264+538+197=999
264+597+138=999
264+598+137=999
267+134+598=999
267+138+594=999
267+143+589=999
267+149+583=999
267+183+549=999
267+189+543=999
267+194+538=999
267+198+534=999
267+534+198=999
267+538+194=999
267+543+189=999
267+549+183=999
267+583+149=999
267+589+143=999
267+594+138=999
267+598+134=999
268+134+597=999
268+137+594=999
268+194+537=999
268+197+534=999
268+534+197=999
268+537+194=999
268+594+137=999
268+597+134=999
269+143+587=999
269+147+583=999
269+183+547=999
269+187+543=999
269+543+187=999
269+547+183=999
269+583+147=999
269+587+143=999
274+136+589=999
274+139+586=999
274+186+539=999
274+189+536=999
274+536+189=999
274+539+186=999
274+586+139=999
274+589+136=999
276+134+589=999
276+139+584=999
276+184+539=999
276+189+534=999
276+534+189=999
276+539+184=999
276+584+139=999
276+589+134=999
279+134+586=999
279+136+584=999
279+184+536=999
279+186+534=999
279+534+186=999
279+536+184=999
279+584+136=999
279+586+134=999
283+147+569=999
283+149+567=999
283+167+549=999
283+169+547=999
283+547+169=999
283+549+167=999
283+567+149=999
283+569+147=999
284+136+579=999
284+139+576=999
284+176+539=999
284+179+536=999
284+536+179=999
284+539+176=999
284+576+139=999
284+579+136=999
286+134+579=999
286+139+574=999
286+174+539=999
286+179+534=999
286+534+179=999
286+539+174=999
286+574+139=999
286+579+134=999
287+143+569=999
287+149+563=999
287+163+549=999
287+169+543=999
287+543+169=999
287+549+163=999
287+563+149=999
287+569+143=999
289+134+576=999
289+136+574=999
289+143+567=999
289+147+563=999
289+163+547=999
289+167+543=999
289+174+536=999
289+176+534=999
289+534+176=999
289+536+174=999
289+543+167=999
289+547+163=999
289+563+147=999
289+567+143=999
289+574+136=999
289+576+134=999
294+137+568=999
294+138+567=999
294+167+538=999
294+168+537=999
294+537+168=999
294+538+167=999
294+567+138=999
294+568+137=999
297+134+568=999
297+138+564=999
297+164+538=999
297+168+534=999
297+534+168=999
297+538+164=999
297+564+138=999
297+568+134=999
298+134+567=999
298+137+564=999
298+164+537=999
298+167+534=999
298+534+167=999
298+537+164=999
298+564+137=999
298+567+134=999
325+176+498=999
325+178+496=999
325+196+478=999
325+198+476=999
325+476+198=999
325+478+196=999
325+496+178=999
325+498+176=999
326+175+498=999
326+178+495=999
326+195+478=999
326+198+475=999
326+475+198=999
326+478+195=999
326+495+178=999
326+498+175=999
328+175+496=999
328+176+495=999
328+195+476=999
328+196+475=999
328+475+196=999
328+476+195=999
328+495+176=999
328+496+175=999
352+168+479=999
352+169+478=999
352+178+469=999
352+179+468=999
352+468+179=999
352+469+178=999
352+478+169=999
352+479+168=999
358+162+479=999
358+169+472=999
358+172+469=999
358+179+462=999
358+462+179=999
358+469+172=999
358+472+169=999
358+479+162=999
359+162+478=999
359+168+472=999
359+172+468=999
359+178+462=999
359+462+178=999
359+468+172=999
359+472+168=999
359+478+162=999
362+158+479=999
362+159+478=999
362+178+459=999
362+179+458=999
362+458+179=999
362+459+178=999
362+478+159=999
362+479+158=999
368+152+479=999
368+159+472=999
368+172+459=999
368+179+452=999
368+452+179=999
368+459+172=999
368+472+159=999
368+479+152=999
369+152+478=999
369+158+472=999
369+172+458=999
369+178+452=999
369+452+178=999
369+458+172=999
369+472+158=999
369+478+152=999
372+158+469=999
372+159+468=999
372+168+459=999
372+169+458=999
372+458+169=999
372+459+168=999
372+468+159=999
372+469+158=999
375+126+498=999
375+128+496=999
375+196+428=999
375+198+426=999
375+426+198=999
375+428+196=999
375+496+128=999
375+498+126=999
376+125+498=999
376+128+495=999
376+195+428=999
376+198+425=999
376+425+198=999
376+428+195=999
376+495+128=999
376+498+125=999
378+125+496=999
378+126+495=999
378+152+469=999
378+159+462=999
378+162+459=999
378+169+452=999
378+195+426=999
378+196+425=999
378+425+196=999
378+426+195=999
378+452+169=999
378+459+162=999
378+462+159=999
378+469+152=999
378+495+126=999
378+496+125=999
379+152+468=999
379+158+462=999
379+162+458=999
379+168+452=999
379+452+168=999
379+458+162=999
379+462+158=999
379+468+152=999
395+126+478=999
395+128+476=999
395+176+428=999
395+178+426=999
395+426+178=999
395+428+176=999
395+476+128=999
395+478+126=999
396+125+478=999
396+128+475=999
396+175+428=999
396+178+425=999
396+425+178=999
396+428+175=999
396+475+128=999
396+478+125=999
398+125+476=999
398+126+475=999
398+175+426=999
398+176+425=999
398+425+176=999
398+426+175=999
398+475+126=999
398+476+125=999
425+176+398=999
425+178+396=999
425+196+378=999
425+198+376=999
425+376+198=999
425+378+196=999
425+396+178=999
425+398+176=999
426+175+398=999
426+178+395=999
426+195+378=999
426+198+375=999
426+375+198=999
426+378+195=999
426+395+178=999
426+398+175=999
428+175+396=999
428+176+395=999
428+195+376=999
428+196+375=999
428+375+196=999
428+376+195=999
428+395+176=999
428+396+175=999
452+168+379=999
452+169+378=999
452+178+369=999
452+179+368=999
452+368+179=999
452+369+178=999
452+378+169=999
452+379+168=999
458+162+379=999
458+169+372=999
458+172+369=999
458+179+362=999
458+362+179=999
458+369+172=999
458+372+169=999
458+379+162=999
459+162+378=999
459+168+372=999
459+172+368=999
459+178+362=999
459+362+178=999
459+368+172=999
459+372+168=999
459+378+162=999
462+158+379=999
462+159+378=999
462+178+359=999
462+179+358=999
462+358+179=999
462+359+178=999
462+378+159=999
462+379+158=999
468+152+379=999
468+159+372=999
468+172+359=999
468+179+352=999
468+352+179=999
468+359+172=999
468+372+159=999
468+379+152=999
469+152+378=999
469+158+372=999
469+172+358=999
469+178+352=999
469+352+178=999
469+358+172=999
469+372+158=999
469+378+152=999
472+158+369=999
472+159+368=999
472+168+359=999
472+169+358=999
472+358+169=999
472+359+168=999
472+368+159=999
472+369+158=999
475+126+398=999
475+128+396=999
475+196+328=999
475+198+326=999
475+326+198=999
475+328+196=999
475+396+128=999
475+398+126=999
476+125+398=999
476+128+395=999
476+195+328=999
476+198+325=999
476+325+198=999
476+328+195=999
476+395+128=999
476+398+125=999
478+125+396=999
478+126+395=999
478+152+369=999
478+159+362=999
478+162+359=999
478+169+352=999
478+195+326=999
478+196+325=999
478+325+196=999
478+326+195=999
478+352+169=999
478+359+162=999
478+362+159=999
478+369+152=999
478+395+126=999
478+396+125=999
479+152+368=999
479+158+362=999
479+162+358=999
479+168+352=999
479+352+168=999
479+358+162=999
479+362+158=999
479+368+152=999
495+126+378=999
495+128+376=999
495+176+328=999
495+178+326=999
495+326+178=999
495+328+176=999
495+376+128=999
495+378+126=999
496+125+378=999
496+128+375=999
496+175+328=999
496+178+325=999
496+325+178=999
496+328+175=999
496+375+128=999
496+378+125=999
498+125+376=999
498+126+375=999
498+175+326=999
498+176+325=999
498+325+176=999
498+326+175=999
498+375+126=999
498+376+125=999
534+167+298=999
534+168+297=999
534+176+289=999
534+179+286=999
534+186+279=999
534+189+276=999
534+197+268=999
534+198+267=999
534+267+198=999
534+268+197=999
534+276+189=999
534+279+186=999
534+286+179=999
534+289+176=999
534+297+168=999
534+298+167=999
536+174+289=999
536+179+284=999
536+184+279=999
536+189+274=999
536+274+189=999
536+279+184=999
536+284+179=999
536+289+174=999
537+164+298=999
537+168+294=999
537+194+268=999
537+198+264=999
537+264+198=999
537+268+194=999
537+294+168=999
537+298+164=999
538+164+297=999
538+167+294=999
538+194+267=999
538+197+264=999
538+264+197=999
538+267+194=999
538+294+167=999
538+297+164=999
539+174+286=999
539+176+284=999
539+184+276=999
539+186+274=999
539+274+186=999
539+276+184=999
539+284+176=999
539+286+174=999
543+167+289=999
543+169+287=999
543+187+269=999
543+189+267=999
543+267+189=999
543+269+187=999
543+287+169=999
543+289+167=999
547+163+289=999
547+169+283=999
547+183+269=999
547+189+263=999
547+263+189=999
547+269+183=999
547+283+169=999
547+289+163=999
549+163+287=999
549+167+283=999
549+183+267=999
549+187+263=999
549+263+187=999
549+267+183=999
549+283+167=999
549+287+163=999
563+147+289=999
563+149+287=999
563+187+249=999
563+189+247=999
563+247+189=999
563+249+187=999
563+287+149=999
563+289+147=999
564+137+298=999
564+138+297=999
564+197+238=999
564+198+237=999
564+237+198=999
564+238+197=999
564+297+138=999
564+298+137=999
567+134+298=999
567+138+294=999
567+143+289=999
567+149+283=999
567+183+249=999
567+189+243=999
567+194+238=999
567+198+234=999
567+234+198=999
567+238+194=999
567+243+189=999
567+249+183=999
567+283+149=999
567+289+143=999
567+294+138=999
567+298+134=999
568+134+297=999
568+137+294=999
568+194+237=999
568+197+234=999
568+234+197=999
568+237+194=999
568+294+137=999
568+297+134=999
569+143+287=999
569+147+283=999
569+183+247=999
569+187+243=999
569+243+187=999
569+247+183=999
569+283+147=999
569+287+143=999
574+136+289=999
574+139+286=999
574+186+239=999
574+189+236=999
574+236+189=999
574+239+186=999
574+286+139=999
574+289+136=999
576+134+289=999
576+139+284=999
576+184+239=999
576+189+234=999
576+234+189=999
576+239+184=999
576+284+139=999
576+289+134=999
579+134+286=999
579+136+284=999
579+184+236=999
579+186+234=999
579+234+186=999
579+236+184=999
579+284+136=999
579+286+134=999
583+147+269=999
583+149+267=999
583+167+249=999
583+169+247=999
583+247+169=999
583+249+167=999
583+267+149=999
583+269+147=999
584+136+279=999
584+139+276=999
584+176+239=999
584+179+236=999
584+236+179=999
584+239+176=999
584+276+139=999
584+279+136=999
586+134+279=999
586+139+274=999
586+174+239=999
586+179+234=999
586+234+179=999
586+239+174=999
586+274+139=999
586+279+134=999
587+143+269=999
587+149+263=999
587+163+249=999
587+169+243=999
587+243+169=999
587+249+163=999
587+263+149=999
587+269+143=999
589+134+276=999
589+136+274=999
589+143+267=999
589+147+263=999
589+163+247=999
589+167+243=999
589+174+236=999
589+176+234=999
589+234+176=999
589+236+174=999
589+243+167=999
589+247+163=999
589+263+147=999
589+267+143=999
589+274+136=999
589+276+134=999
594+137+268=999
594+138+267=999
594+167+238=999
594+168+237=999
594+237+168=999
594+238+167=999
594+267+138=999
594+268+137=999
597+134+268=999
597+138+264=999
597+164+238=999
597+168+234=999
597+234+168=999
597+238+164=999
597+264+138=999
597+268+134=999
598+134+267=999
598+137+264=999
598+164+237=999
598+167+234=999
598+234+167=999
598+237+164=999
598+264+137=999
598+267+134=999
may I ask for the code used to find these combinations? thanks.
how many problems you have that add up to 999 is that your love number
math and 999 sitting in a tree KISSING 1st comes the love 2nd comes the marrige 3rd comes 889 in the little carrige
598+267+134=999
are you in love with 999? dude, you’re weird. shut up dummy.
yea
huh
YEAH!!!!!!!
That’s a lot of 999 gee -_-
too much ‘___’
hahahahahahahahahahaha
stop giving out the answer>.<
chicken wing chicken wing hot dog and baloney chilling with my homie hot dog and baloney
hehe boi
u stink
you forgot 999+0+0=999
597+238+164=999
SHUT UP DUMMY I”M TIRED OF 999!
Somebody call 911 he or she cursed
564+121+311=996
bruh
should be 247+563+180
how many problems do you have
gee, do not say that!
why
stop changing your name!
why>.<
I HATE YOU, ZOEY!!!!
why
The m word is so racist. She said the racist word for Chinese people.
cotcotcotcotcotcotcotocotocotcotocotcotcotcotcotcotcotcotcotcotcotcotcotcotcotcotcotcotcotcotcotcotcotcotcotcotcotcotocotcotcotcotcotcotcotocotcot
what are you saying.
hahahahahahahahahahahahahahahahahahahahahahahahahahahahaha
gee,you are so mean to zoey
yeah so mean I A.G.R.E.E
589 + 276 +134 = 999
I gave this to my 7th grade math team after seeing in it the Open Middle Math book. I made it a three step investigation. Fist was find the largest sum, then the smallest then get as close to 999. The loved it, especially the third challenge. It lead us into some counting theory discussion regarding permutations. One kid even observed that the ones column adds to 19, the tens adds to 18 and the hundreds adds to 8. They searched for different solutions that each had 216 permutations. And they are at 5 as of now.
I love how something that seems so simple can become so engaging and complex.
EEEEEEEEEEEEEEEEEEEEEWWWWWWWWWWWWWWWWWWWWWWWWW, are you engaged?
who are you in love with?
Hope Levio, you’re weird
oh gash
One would be 365 + 426 + 198
One would be he he boi
ok
Does anyone have a way to prove that getting exactly 1000 is impossible using only elementary math?
Thanks.
Thanks who? Dude, you’re weird.
uno reverse
haha
Uno Reverse Car
What
shut up
UNO reverse means not reverse since it’s Reverse
SHUT UP
stop!!!!!!!!!!!!!
why
COTY COT COT
you stink
COTCOTCOTCOTCOTCOTCOTCOTCOTCOTCOT
Cot cot cot cot cot cot cot cot Coty
not again!
omg!!!!!!!!!!
youwertyuik
stop!!!!!!
BUNGGEEEEEEe
bungee???????!!!!!!!!!?????????
Yeah bungee man!
stop it dumbheds!!!!!
hey who are you calling dumb watch your mouth
guys the ice cream truck is leaving in 10 secs!!!
10 9 8 7 6 5 4 3 2 1 ZOOOOOOOOOOOOOOOOOOOOOOOOM!
shut up
whyyyyyyyyyyyyyyyyyyyyyyyy you silly chicken
shut up , you DUMBHEAD!!!!!
Im NOT A CHIIIIIIIIIIIIIIIICCCCCCCCCCKKKKKKKKKKKKEEEEEEEENNNNNNNN!!!!!!!!!!!
no, you shut up
Uno reverse
nope card
hi i’m komma’s sis!
shut up
bruhhhhhhhhhhhhhhhhhhhhhh
oink!!!!!!!!!!!
are you just maggie?
hahahahahahahahahahahahahahahahahahahahahahahahahahahahahahahah
i know its you, maggie!
yeah you xot cot
poker face by Lady gaga
poop your pants
is a poker face somebody with pokers on their face?
LLLLLLLLLLLLLLLLLLLLLLLLLLLLLLAAAAAAAAAAAAAAAAAAAAAADDDDDDDDDDDDDDDDDDYYYYYYYYYYYYYYYYYY GGGGGGGGGGGGGGGGGGAAAAAAAAAAAAAAAAAAAAGGGGGGGGGGGGGGGGGGAAAAAAAAAAAAAAAAA
huhuhuuhuhuh??????????????????
pigoink
stoopid
vomit 21o
where your underwhere up & down shake your butt round & round
rip your underwear and shake your butt in front of mrs. ramirez
bbbbbbruh
beep boop!
v87
YYYYYYYYYYYOOOOOOOOOO MMMMMMOOOOOOOMMMMMMMNMMMMMMMAAAAAAAA
ugh
huh?
shut up
yo sissy
you should be ashamed of yourself!
hey yo sissy
did i miss anything?
you missed the part when poop went on Komma’s head
poop pee fart butt butthole toilet
yes ??? you missed the part when a flying pig came by
i poop alot
you pee a lot
yo sissy
nope nope nope poop poop poop
bro
grrrrr
ivr43uh
caca
poop
You peed on your hand yesterday. HEHEHEHEHE
I bet your mama so fat that even Dora can’t explore her
Dora the Poop Explorer
your turn!
yo mama hates you cause your mouth to big and you never brush your teeth
yo mama so dumb she said a beanstalk is a secret agent
yo mama so dumb iq -100
oh yeah? yo mama so ugly hello kitty said goodbye
yo komma your butt so big even ian kissed it
ur dead
You cot cotted
YEAH!!!! YOU ARE DEAD!!!!!!!
oh yeah!
I hate you qwegh!
so do i!
SSSSSSSSSSSHHHHHHHHHHHHHHHUUUUUUUUUUUUUUUUTTTTTTTTTTTTT UUUUUUUUUUUUPPPPPPPPPPPPPPP!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!
you cot cot gorilla snickerdoodle overweighted toaster that only cookes lasgana!
delete it right now!
not trueeeeeeeeeee
ok uh… yo mama so ugly that she was mistaken for a gorilla
Yo sissy so fat she was mistaken for a pig
mora the expoola
yo mama so big things orbit her
Coty CotCot
hey people depot
BIRDS CAN FLY!
peoplpeoplpeoplpeoplpeople
Hey everybody, this is my secret. BIRDS CAN FLY!
;0
Coty Cot
hahahahahahahahahahaha
99999999999999999999999999999999999
U R CURSED!
ask miss vashee if we can do prodigy
why?
me stink
U STINK!
you bannana doughnut lollypop that dispenses gronila bars!
IAN LICKS HIS FEET!
tutututututututututututututututututututututututututut
thy has bean kelped quivered for mallinia
4 your information, eye do not understand u
what
huh?
huhuhuhuhuhuhuhuhuh???
>o[]< lol 0_o
nooooooo
bootycheek
I M dumb
i m not
0+0+999+
okeydoekey
you are farty’s face
yo mama